The Option Greeks Explained
The Option Greeks are several statistical quantities that we use for measuring sensitivity in options trading.
After reading this short introduction, you’ll have learned the following:
- What the Option Greeks are and why they are used.
- How options traders calculate the Greeks.
- How the Option Greeks can be used for assessing risk.
- How you make better options trading decisions based on this extra knowledge.
What are the Option Greeks?
The Option Greeks measure the sensitivity of an option in respect of the underlying asset. The different factors that influence the value of an option can be quantified. Five key Greeks exist. Delta, Gamma, Theta, Vega, and Rho.
What is the Motivation behind the Option Greeks?
Various factors can have an impact on options pricing. These factors can be expressed by comparable values. They are called the Option Greeks due to the Greek letters that are used for those values. The Greeks help options traders with picking the right contracts at the right time. If you trade options without basic knowledge of the Greeks, you may miss critical information. It’s like being blindfolded and making decisions based on fewer data.


How we calculate the Option Greeks is of less importance. More important is to learn what information the Greeks incorporate and how we can employ them in our daily trading activities. An options trader doesn’t need to be a mathematical genius. The actual statistical formulas are of less importance. But you need to understand if the option premium is low or high and how it affects your strategies and decisions.
With the following tutorial, you’ll be able to make a sensitivity analysis and assess the potential risk and reward of your strategy for any options contract. You can use the Greeks to
- evaluate open positions
- buy the right option contracts for your strategy
- determine option sensitivity in general
With the Greeks, options trading becomes more analytical. We can make better decisions that match our trading strategies. When we start to go into the details, in the beginning, you might feel overwhelmed by the complex formulas and Greek letters. But don’t worry! Understanding what these numbers and letters mean is more important than the equations.
How are the Greeks calculated?
We focus on the five Greeks with the highest relevance.
| Name | Value depends on | Relative to |
|---|---|---|
| Delta | Option price | Underlying asset |
| Gamma | Delta | Underlying asset |
| Vega | Option price | Implied volatility |
| Theta | Option price | Time until expiration |
| Rho | Option price | Interest rate |
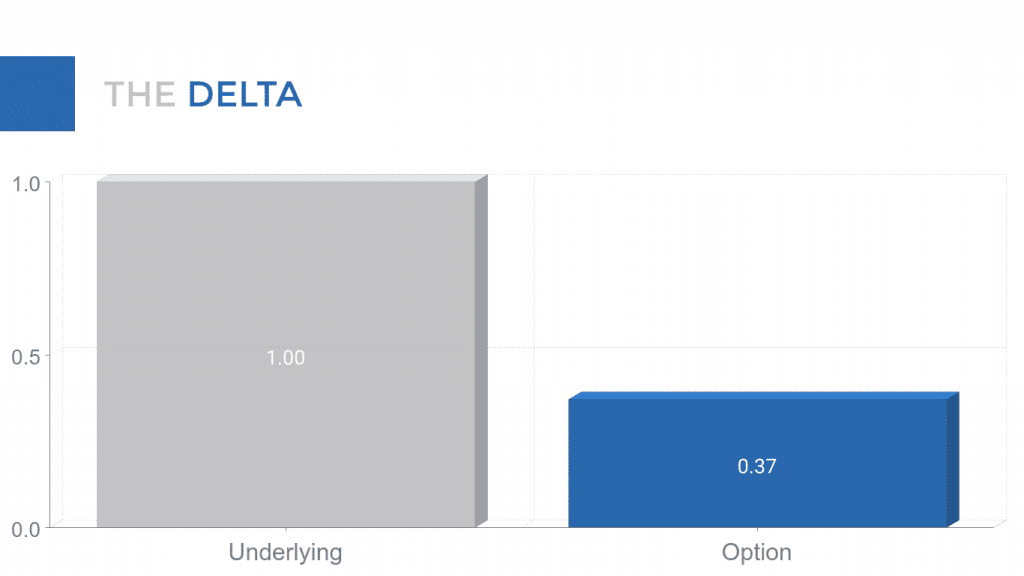
Delta
Delta (Δ) calculates the sensitivity of the option price relative to the underlying asset. If the value of the underlying increases or decreases by $1, then the option price increases or decreases by the amount Δ.

The mathematical formula for Delta is:
Δ = ∂ V / ∂ S
where ∂ is the first derivative, V the option price, and S the value of the underlying. Here, we use the first derivatives because they measure how the rate of a variable will change over a given period. Delta is a decimal number between -1.00 and 1.00. If the Delta is between -1.00 and 0, then it’s a Put option. Likewise, an option with a Delta between 0 and 1 is a Call Option.
Using Delta
For comparing options
There’s a direct connection between an option’s value and its Delta. The deeper an option is in-the-money, the closer its Deltas is to 1 or -1.
For hedging and calculating position sizes
Delta also represents a hedge ratio. When we know the current Delta, then we can hedge our underlying asset and calculate the right position size easily. All we have to do is multiply the value of our asset with the Delta and then buy Call or Put options in the computed number.
Gamma
Gamma (Γ) measures how much Delta changes relative to the price of the underlying asset.
One could think that there’s a linear coherence between the Delta and the underlying. But we have learned that in-the-money and out-of-the-money options react with different sensitivity to price changes. Therefore, the Delta and the option price aren’t linear.

The mathematical formula for Gamma is:
Γ = ∂ Δ / ∂ S
where ∂ is the first derivative, Δ the Delta and S the price of the underlying. Quickly explained, when the price of the underlying changes by $1.00, then the Delta changes by the amount Gamma represents. Again, the Gamma is negative for Put options and positive for Call options.
Using Gamma
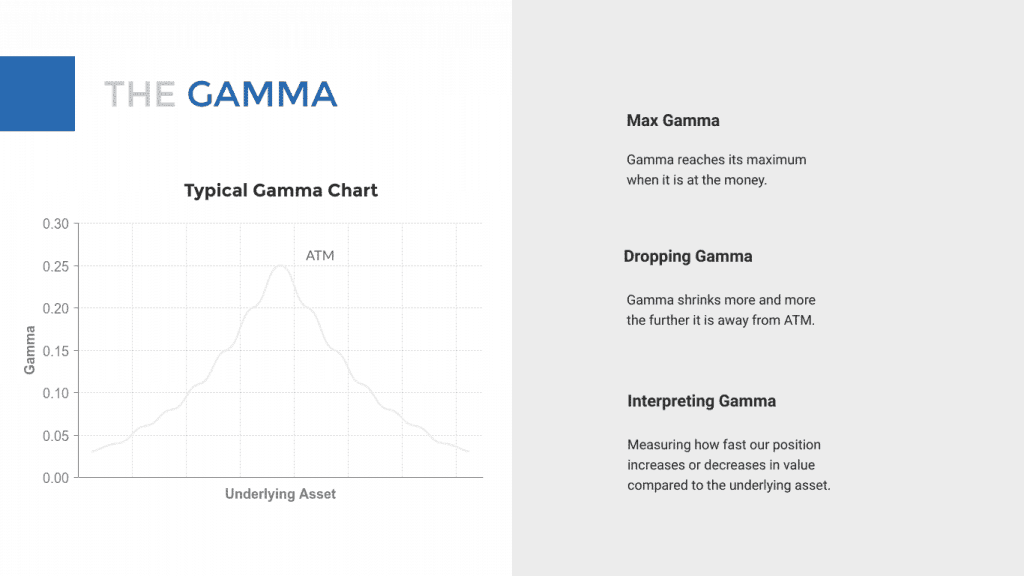
With Gamma, we can assess how the option’s price will move when the asset starts to move. Gamma helps options traders to analyze the current situation of an option. Is the option in-the-money or out-of-the-money?
Analyzing the moneyness of an option
The Gamma reaches it’s maximum when it is at-the-money. On the other hand, Gamma starts to decrease the more an option is in-the-money or out-of-the-money. That’s precisely the reason why many traders buy options at-the-money. Thus, the option will participate more from price changes of the underlying. We usually try to find an option with a Gamma close to 1.00 or -1.00.
Vega
Vega (ν) determines the sensitivity of the option price relative to the implied volatility (IV) of the underlying. If the IV rises by one percent, then the option value increases by the value of Vega. Calls have positive Vega while Puts have negative Vega.
ν = ∂ V / ∂ σ
where ∂ is the first derivative, V the option’s price and σ the volatility of the underlying asset. Vega is represented by a fixed amount rather than a percentage. A higher vega results in higher option value. This applies to both Call and Put options.
Using Vega
Analyzing the influence of implied volatility on the option’s value
With Vega, we can estimate how much shifts in the implied volatility will affect the options price. It should be noted that Vega decreases over time. Hence, it needs to be monitored periodically.
Picking competitive spreads
An option has a competitive spread when the amount of Vega is greater than the option’s bid-ask spread. This can be significant for hedging strategies.
Theta
Theta (θ) measures the sensitivity of the option price relative to its time to expiration. When the time to expiration diminishes by one day, then the price changes by the current Theta. That’s why we can say that Theta estimates the daily time decay.
θ = – ∂ V / ∂ τ
where ∂ is the first derivative, V the option’s price and τ the time until maturity. In most cases, the time value shrinks until it reaches 0 at the time of expiration. That’s why Theta is negative for most options. Exceptions can be found in European options.
Using Theta
Speculation on small movements in a short period of time
For this strategy, we need to find options with sufficiently low theta values. This is due to the fact that losses through time decay (extrinsic value) need to be smaller than gains in intrinsic value.
Profiting from sideways markets
That’s more or less the opposite strategy. You plan to have financial gains from time decay.
For both strategies, it’s essential to analyze Theta.
Rho
Rho (ρ) quantifies the sensitivity of the option price relative to a benchmark interest rate. The option price changes by Rho when the corresponding interest rate moves 1%. Generally, the Rho Greek has the least impact on options trading. For completeness, we don’t leave it out, though.
ρ = ∂ V / ∂ r
where ∂ is the first derivative, V the option’s price, and r the benchmark interest rate. When the benchmark interest rises, then Call options increase in price.